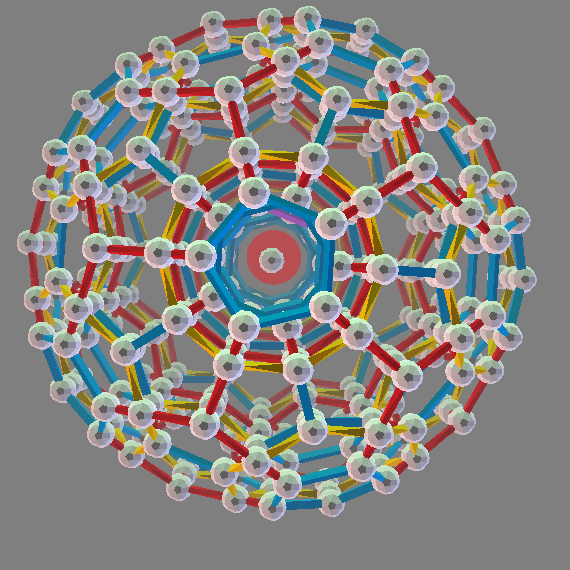
Dave Van Dyke of Z Axis Graphics, who generated this lovely Dodecadodecahedron animation video, just generously contributed a free companion Dodecadodecahedron screen saver (Windows only; In Windows it wants to install in C:\Windows\system32\); enjoy!!! Dave’s comment about the screen saver gift: “…just thought it would be fun.” Thanks, Dave!!!
Notes from the animator (Dave Van Dyke):
The dodecahedron was created and animated using 3DS Max. If you intend to animate the “P” and “Q” parameters of the Hedra you must use the Edit Mesh modifier. The Edit Poly modifier will not work. The nice Glow effect is an option in the Mental Ray renderer found under the Renderer Tab. The frame is formed by making a copy of the dodecahedron and applying the Lattice modifier. The material assigned to the dodecahedron is a Multi/subObject material. The material for the star faces and the remainder of the dodecadodecahedron are the same. Having two materials that are the same allows you to take control of the transparency of one selection set of polygons leaving the others unaffected.

Update (16July2011): Dave has again contributed another interesting screensaver. This one is an organic-looking simulation of magnetic particles streaming around the magnetic lines of force defined by a torus. Thanks again, Dave!